1. For the case of vector potential derived for the solenoid show that the expression satisfies Coulomb gauge condition.
In cylindrical coordinates 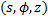
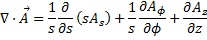
Since 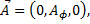 we only have to consider the second term. As
we only have to consider the second term. As 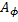 is independent of Φ the divergence is zero.
is independent of Φ the divergence is zero.
2. Find the vector potential inside a cylindrical wire of radius R.
The field inside a cylindrical wire carrying a current in the z direction is given by
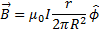
Since the magnetic field is along the azimuthal direction, we have on equating it with the azimuthal component of the vector potential,
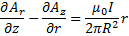
3. A charge q is moving slowly with a uniform velocity 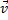 .Obtain an expression for the vector potential and the magnetic field at a distance r from it.
.Obtain an expression for the vector potential and the magnetic field at a distance r from it.
From Tutorial problem 2 it follows that the contribution to the field due to two straight sections is zero. The smaller semicircle section gives a field into the page while the bigger semicircle gives a field out of the page. The net field is (into the page)
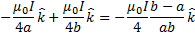
![]()
![]() we only have to consider the second term. As
we only have to consider the second term. As ![]() is independent of Φ the divergence is zero.
is independent of Φ the divergence is zero. ![]() .Obtain an expression for the vector potential and the magnetic field at a distance r from it.
.Obtain an expression for the vector potential and the magnetic field at a distance r from it.